Introduction
Just as for the Two Factor ANOVA there are both with replication and without replication versions of Three-Factor ANOVA. In ANOVA with More Than Two Factors and Three-Factor ANOVA Analysis we dealt with data with replications.
In Two Factor ANOVA without Replication, there is no interaction factor, while in the Three-Factor ANOVA without Replication there is no interaction of the three factors, but there are pairwise interactions. Thus, we have two possibilities: (1) pairwise interactions are separated and (2) pairwise interactions are included in the error.
Example
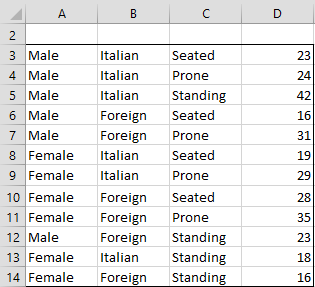
Example 1: Perform Three-Factor ANOVA on the data in Figure 1 using the Real Statistics Three-Factor ANOVA data analysis tool.
Figure 1 – Three-Factor ANOVA data
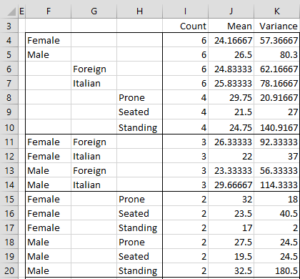
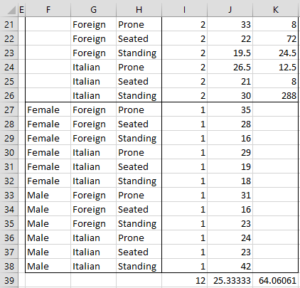
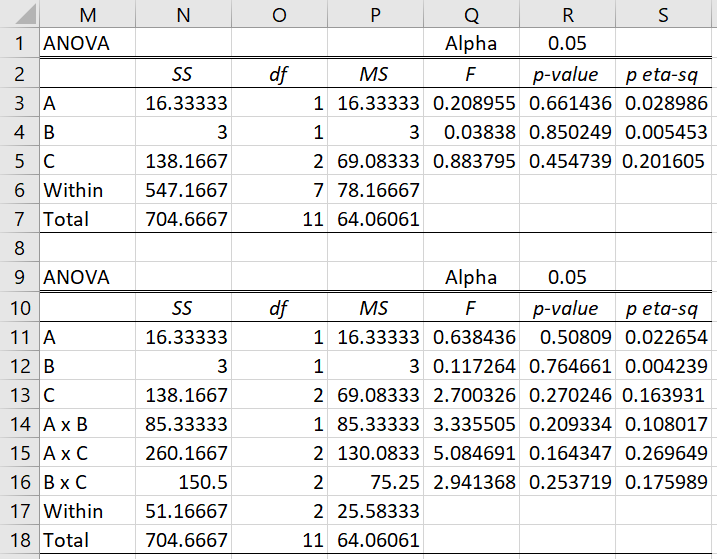
Note that there are no interactions among the three factors in the data in Figure 1. The Three-Factor ANOVA data analysis tool described in Three Factor ANOVA Analysis can be used with A3:D14 as the Input Range. The descriptive data output is shown in Figure 2 and the two forms of the ANOVA analysis are shown in Figure 3.
Figure 2 – Three-Factor ANOVA descriptive statistics
Note that in the first ANOVA output in Figure 2, SSW (in cell N6) is calculated using the formula =N7-N5-N4-N3 (and similarly for the dfW term). All the other cells are calculated in the usual way. In the second ANOVA output the A × B, B × C and A × C are calculated as in ANOVA with replication and only the A × B × C is omitted. Here SSW (cell N17) is calculated using the formula =N18-N16-N15-N14-N13-N12-N11.
Figure 3 – Three-Factor ANOVA output
Examples Workbook
Click here to download the Excel workbook with the examples described on this webpage.
References
Bao, J. (2021) Two-, three-, and four-way ANOVA
https://sites.pitt.edu/~jub69/material/material/stat1301/Lec/SAS-ANOVA-2.pdf
Laerd (2024) Three-way ANOVA in SPSS statistics
https://statistics.laerd.com/spss-tutorials/three-way-anova-using-spss-statistics.php
Meier, L. (2023) Factorial treatment structure. ANOVA and mixed models: a short introduction using R.
https://people.math.ethz.ch/~meier/teaching/anova/index.html
I have 7 years data of two companies on 10 different segments, Can you suggest which test is suitable for test the significance difference ?
First things first. When you refer to a significant “difference”, you clearly mean a difference between A and B. What are A and B in this context? This is a non-trivial question.
Charles
HI Mrs. Charles
here I want to do three factorial research based on my title but, I am not clear with the treatment combination of the three factors. so, please try show me some examples..
Best regards
Safesa
Hi Safesa,
Can you explain further what you are not clear about? Do you want clarification about Three-Factor ANOVA without Replication or Three-Factor ANOVA with Replication?
Charles
Hi!
Thank you so much for your input.
I have a question I was hoping to be answered in this page…
How can I interpret the coefficients (with more than 2, lets say 3 levels as shown above) from a multiple linear regression model?
Thank you,
Hi Raj,
I had never thought about this before, and so don’t know the answer to your question. Perhaps someone else from the community can answer your question.
Charles
please send the excel template
Hello Sadikul,
I am not sure what you mean by the excel template, but if you are looking for the worksheets described on this website go to
Real Statistics Examples Workbooks
Charles