Properties
The pdf of the Logistic distribution at location parameter µ and scale parameter β is
where β > 0. The cdf is
The inverse of the logistic distribution is
The standard Gumbel distribution is the case where μ = 0 and β = 1.
Key statistical properties of the Logistic distribution are shown in Figure 1.
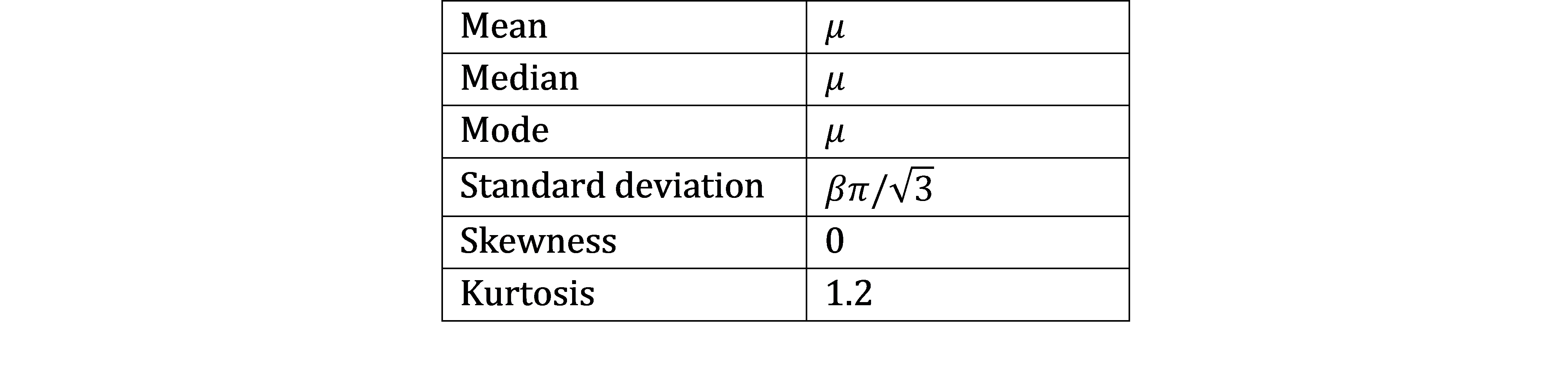Figure 1 – Statistical properties of the Logistic distribution
Graphs
Figure 2 shows a graph of the Logistic distribution for different values of μ and β.
Figure 2 – Chart of Logistic distribution
Worksheet Functions
Real Statistics Functions: The Real Statistics Resource Pack provides the following functions for the Logistic distribution.
LOGISTIC_DIST(x, μ, β, cum) = the pdf of the Logistic distribution f(x) when cum = FALSE and the corresponding cumulative distribution function F(x) when cum = TRUE.
LOGISTIC_INV(p, μ, β) = the inverse of the Logistic distribution at p
Examples Workbook
Click here to download the Excel workbook with the examples described on this webpage.
References
Wikipedia (2020) Logistic distribution
https://en.wikipedia.org/wiki/Logistic_distribution
Hastings, N., Peacock, B. (2011) Statistical distributions. 4th Ed, Wiley
https://www.wiley.com/en-us/Statistical+Distributions%2C+4th+Edition-p-9780470390634
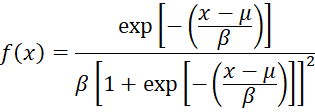
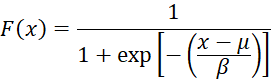
After downloading the resource pack on my android, I am still getting an error for using the function LOGISTIC_DIST. I believe this particular function is still not supported by the downloaded version.
Hello Adebayo,
I just checked and LOGISTIC_DIST works on my computer.
You say that you downloaded the Real Statistics Resource Pack on your android. Did you also install the resource pack? I ask since I don’t think the resource pack works on android. What do you see when you enter the formula =VER() in any cell?
Charles