Functions, polynomials, limits and graphs

Functions

A function is a mapping between two sets, called the domain and the range, where for every value in the domain there is a unique value in the range assigned by the function. Generally, functions are defined by some formula; for example f(x) = x² is the function that maps values of x into their square. The mapping is between all numbers (the domain) and non-negative numbers (the range).

Polynomials

A function is polynomial if it has the form

for some non-negative integer n (called the degree of the polynomial) and some constants a₀, a₁, …, a_n where a_n ≠ 0 (unless n = 0). The function is linear if n = 1 and quadratic if n = 2.

Limits

We often use limit notation such as

as a shorthand for as x gets larger the value of the function f(x) approaches the value a. Some examples for various values of f(x) are as follows:

We can also evaluate the limit of a function when x approaches some other value. For example, in the following example as x gets close to 0 the value of the function approaches 1.

We can also use the limit notation with series. If x_n = 1 – 1/n where n is a positive integer then

since the series , , , ,  … converges to 1.

Graphs

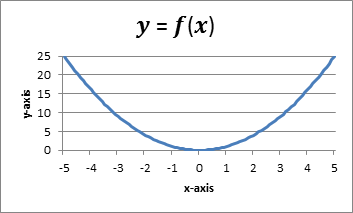
Often we use a graph to show the relationship between x and f(x). A graph consists of all the points (x,y) in what is called the xy plane where x is in the domain of f and y = f(x). The graph is drawn in a rectangular grid defined by the x-axis (drawn horizontally) and the y-axis (drawn vertically) meeting at a point (0, 0) call the origin. In particular, the graph of y = f(x) for f(x) = x² is given in Figure 1.

Figure 1 – Graph of  y = f(x) where f(x) = x²

A line is a set of points (x, y) such that y = bx + a for some constants a and b. b is called the slope of the line and a is called the y-intercept.