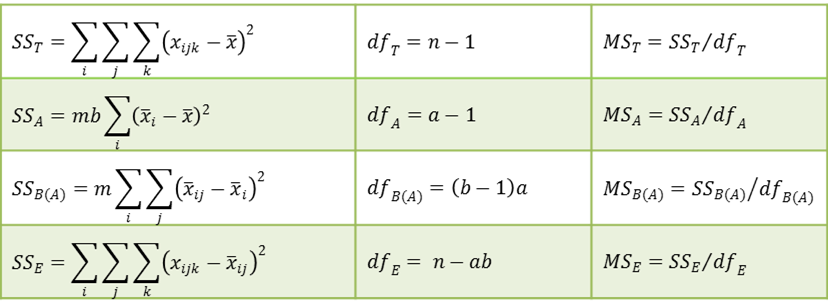Fixed Factor Nested ANOVA

The structural model for a nested design with two fixed factors is given by the formula

Here μ, α_i  and β_j(i) are constants where β_j(i) is the effect of the jth level in Factor B nested within the ith level of Factor A.

We assume that for all i, j and k, the ε_ijk are pairwise independent and

We can conclude that for all i, j and k, the x_ijk are independent and normally distributed with mean

and variance

We also have the following estimators

The definitions of  SS_A, SS_B, SS_AB, SS_E, SS_T and similarly for the corresponding MS and df terms are exactly the same as defined for fixed factors crossed designs (see Definition 2 of Two Factor ANOVA with Replication). SS_B(A) is defined by adding the SS_B and SS_AB terms and df_B(A) is defined by adding the df_B and df_AB terms. This results in the following:

We also have

The null and alternative hypotheses are as follows. There is no interaction between factors A and B.

Test Desired	Null H0	Alt Hyp H1	Statistical Test
Effect of factor A	αi = 0 for all i	αi ≠ 0 for some i	MSA/MSE ∼ F(dfA,dfE)
Effect of B(A)	βj = 0 for all j	βj ≠ 0 for some j	MSB(A)/MSE ∼ F(dfB(A),dfE)